Question

clinical trial was conducted to test the effectiveness of a drug for treating insomnia in older subjects. before treatment 19 subjects had a mean wake time of 100.0 min after treatment the 19 subjects had a mean wake time of 71.6 min and a standard deviation of 20.4 min assume that the 19 sample value appears to be from a normally distributed population and construct a 99% confidence interval estimate of the mean wake time for a population with drug treatment what does the result suggest about the wake time of 100.0 min before the treatment does the drug appears to be effective

Answer 1

We have to calculate a 99% confidence interval for the mean.

The population standard deviation is not known, so we have to estimate it from the sample standard deviation and use a t-students distribution to calculate the critical value.

The sample mean is M=71.6.

The sample size is N=19.

When σ is not known, s divided by the square root of N is used as an estimate of σM:

`s_M=(s)/(√(N))=(20.4)/(√(19))=(20.4)/(4.359)=4.68`

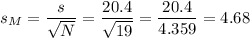
The degrees of freedom for this sample size are:

`df=n-1=19-1=18`

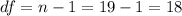
The t-value for a 99% confidence interval and 18 degrees of freedom is t=2.878.

The margin of error (MOE) can be calculated as:

`MOE=t\cdot s_M=2.878\cdot4.68=13.471`

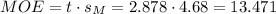
Then, the lower and upper bounds of the confidence interval are:

`\begin{gathered} LL=M-t\cdot s_M=71.6-13.471=58.129 \\ UL=M+t\cdot s_M=71.6+13.471=85.071 \end{gathered}`

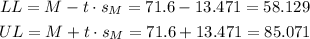
The 99% confidence interval for the mean is (58.129, 85.071). This interval does not include the value 100, so we can conclude that there is statistical evidence that the treatment reduces the mean wake time.