SOLUTION
The figure below would help in answering the question
Let's get the slopes of the line for company G and company H
Slope m is given as

For company G, we have slope as
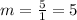
For Company H, we have
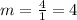
From the graph
Cab fare for 1 mile with company G is $7
Cab fare for 10 miles with company H is?
To get this we need to get the equation of the line H
From

Now substituting x for 10 in the equation, we have
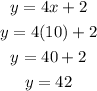
Hence the cab fare for 10 miles with Company H is $42
The rate charge per mile by Company G is the slope we got as 5.
Hence the answer is $5 per mile
The rate charge per mile by Company H is the slope we got as 4.
Hence the answer is $4 per mile