Question

You deposit $3000 each year into an account earning 8% interest compounded annually. How much will youhave in the account in 20 years?

Answer 1

The rule of the compounded interest is

`A=P(\lbrack(1+(r)/(n))^(nt)-1\rbrack)/((r)/(n))`

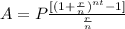
A is the final amount

P is the amount each year

r is the interest rate in decimal

t is the time

n is the number of periods per year

Since you deposit $3000 each year, then

P = 3000

Since the annual rate is 8%, then

r = 8/100 = 0.08

n = 1

Since the time is 20 years, then

t = 20

Substitute them in the rule above to find A

`\begin{gathered} A=(3000\lbrack(1+(0.08)/(1))^(1(20))-1\rbrack)/((0.08)/(1)) \\ A=(3000\lbrack(1.08)^(20)-1\rbrack)/(0.08) \\ A=137285.8929 \end{gathered}`

You will earn $137 285.8929 after 20 years