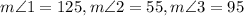Given:
Given the diagram
Required: The measure of angles 1, 2 and 3.
Step-by-step explanation:
The sum of interior angles of a triangle is 180 degrees. So,
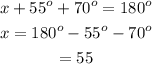
The angles x and 1 are supplementary angles, that is, the sum of angles 1 and x gives 180 degrees.
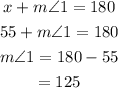
The angle 2 is the opposite angle of 55 degrees. Hence angle 2 equals 55 degrees, that is,
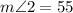
Angle y and 150 degrees are supplementary angles. So,
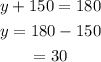
The sum of the interior angles of a triangle equals 180 degrees.
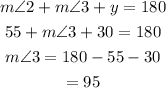
Final Answer: