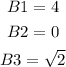Question

Write an equation of each circle described below. Show work! (Hint: find the coordinates of the center first)Given a circle with (5, 1) and (3,-1) as the endpoints of the diameter.(x − B1)² + (y - B2)² = (B3)²B1=B2=B3=Blank 1:Blank 2:Blank 3:Submit

Answer 1

Given:

It is given that a circle is represented by two end points (5,1) and (3,-1).

Find:

we have to find the equation of the circle, radius and center of the circle using end points.

Step-by-step explanation:

The circle represented by two end points (5,1) and (3,-1) is drawn as

The diameter of the circle is

`d=√((5-3)^2+(1-(-1))^2)=√(4+4)=√(8)=2√(2)`

Therefore radius of the circle is

`B3=(d)/(2)=(2√(2))/(2)=√(2)`

The center of the circle is

`(B1,B2)=((5+3)/(2),(1-1)/(2))=((8)/(2),(0)/(2))=(4,0)`

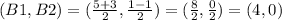
Therefore, the equation of the circle is

`(x-4)^2+(y-0)^2=(√(2))^2`

where,

`\begin{gathered} B1=4 \\ B2=0 \\ B3=√(2) \end{gathered}`