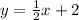Question

What is the equation

Answer 1

In the graph you can see that the line passes through 2 points (-4,0) and (0,2). With them you can obtain the equation of the line. First you find the slope of the line with the following equation

`\begin{gathered} m=(y_2-y_1)/(x_2-x_1) \\ \text{Where} \\ m\colon\text{ Slope of the line} \\ (x_1,y_1)\colon\text{ Coordinates of first point }on\text{ the line} \\ (x_2,y_2)\colon\text{ Coordinates of second point }on\text{ the line} \end{gathered}`

So you have,

`\begin{gathered} (x_1,y_1)=(-4,0) \\ (x_2,y_2)=(0,2) \\ m=(y_2-y_1)/(x_2-x_1) \\ m=(2-0)/(0-(-4)) \\ m=(2)/(4)=(1)/(2) \end{gathered}`

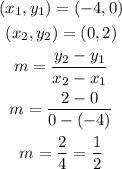
Now, with the point slope equation you can obtain the equation of the line

`\begin{gathered} y-y_1=m(x_{}-x_1) \\ y-0=(1)/(2)(x-(-4)) \\ y=(1)/(2)(x+4) \\ y=(1)/(2)x+(1)/(2)\cdot4 \\ y=(1)/(2)x+(4)/(2) \\ y=(1)/(2)x+2 \end{gathered}`

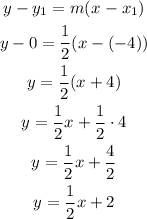
Therefore, the equation of the line is

`y=(1)/(2)x+2`