Question

Passing through (- 4,-7) and (1,3) What is the equation of the line in point-slope form

Answer 1

To calculate the equation of the line passing through the points ( -4. -7) and (1, 3):

`\begin{gathered} \text{ Equation of the line can be calculated by the formula } \\ y-y_1=m(x-x_1) \end{gathered}`

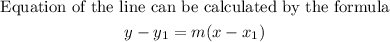
where m = slope

(x1, y1) = any of the points given; say points (-4, -7). That is x1= -4, y1 = -7

To calculate the slope, m:

`\begin{gathered} \text{ m = }\frac{y_2-y_{1_{}}}{x_2-x_1}_{} \\ \text{ where }(x_2,y_2)\text{ = (1, 3)} \\ m\text{ = }\frac{3\text{ - (-7)}}{1-(-4)} \\ m=\text{ }(3+7)/(1+4)\text{ = }(10)/(5) \\ m=2 \end{gathered}`
`\begin{gathered} \text{ substituting m = 2, x}_1=-4,y_1=-7\text{ into the formula }y-y_1=m(x-x_1) \\ we\text{ have} \\ y-(-7)=2(x-(-4)\text{ )} \\ y+7=\text{ 2(x+ 4)} \end{gathered}`

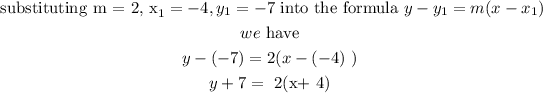
The equation of the line in point slope form is y + 7 = 2(x + 4)