Question

(a) Find an angle between 0° and 360° that is coterminal with 600°.(b) Find an angle between 0 and 2n that is coterminal withЗп2

Answer 1

Coterminal Angles are angles that share the same initial side and terminal sides.

Finding coterminal angles is as simple as adding or subtracting 360° or 2π to each angle, depending on whether the given angle is in degrees or radians.

QUESTION A

The angle is given as 600°.

To find the coterminal angle between 0° and 360°, we subtract 360° from the angle.

Therefore,

`\text{Coterminal angle = 600 - 360 = 240}\degree`

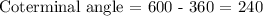
The coterminal angle is 240°.

QUESTION B

The angle is given as

`-(3\pi)/(2)`

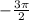
To get an angle between 0 and 2π, we will add 2π to it.

Hence, we have

`\begin{gathered} \text{Coterminal angle = 2}\pi-(3\pi)/(2) \\ =(\pi)/(2) \end{gathered}`

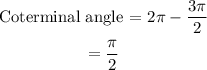
The coterminal angle is π/2.