Question

Hello! I'm hitting a bit of a snag on this. I think I'm reading it too many times

Answer 1

The solution:

Given:

`\begin{gathered} \text{ A sphere of radius 4m.} \\ \\ A\text{ cube of side 6.45m} \end{gathered}`

Required:

To compare the volume and area of bot shapes.

The Sphere:

`\begin{gathered} Area=4\pi r^2=4(4)^2\pi=64\pi=201.062m^2 \\ \\ Volume=(4)/(3)\pi r^3=(4)/(3)*\pi*4^3=268.083m^3 \end{gathered}`

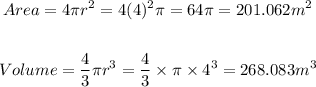
The Cube:

`\begin{gathered} Area=6s^2=6*6.45^2=249.615m^2 \\ \\ Volume=s^3=6.45^3=268.336m^3 \end{gathered}`

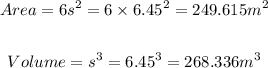
Clearly, we can see that:

Both shapes have approximately the same volume.

But the cube has a greater volume than that of the sphere.

Therefore, the correct answer is [option 4]