Let's call FC the fixed cost for production and VC the variable cost per item.
The company believes they can sell 2,500 items at $245 each.
Production costs:
For producing 2,500 items, the company has to spend (total cost, TC):
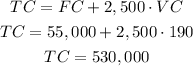
Sells:
Now, company sells eacho of the 2,500 items at $245, so, the company income (I) is:
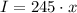
where x is the number of items sold.
Break-even point:
This point is reached when company can recover the money they spend (TC). So, we have the following eaquation to solve:
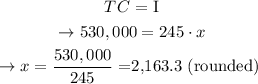
Since company can not sell fractions of items, they have to sell 2,164 items to take back the money they invested.
So, "None of these choices are correct".