Question

Use intercepts to graph the line described by the equation.4y =5x +20

Answer 1

We have to graph the equation 4y=5x+20 using the intercepts.

First we are going to find the y-intercept of the line, to do this we have to remember that the line intercepts the y-axis when x=0. Plugging this value of x in the equation we have

`\begin{gathered} 4y=5(0)+20 \\ 4y=20 \\ y=(20)/(4) \\ y=5 \end{gathered}`

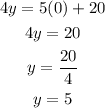
Hence the y-intercept is 5. This represents the point (0,5).

Now we draw this point on the graph:

Now we have to find the x-intercept of the line, this happens when y=0. Plugging this value of y in the equation we have that

`\begin{gathered} 4(0)=5x+20 \\ 0=5x+20 \\ 5x=-20 \\ x=-(20)/(5) \\ x=-4 \end{gathered}`

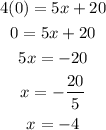
Then, the x-intercept is -4. This represents the point (-4,0).

Drawing this point on the graph we have.

Now we only connect the points with a straight line:

That's the graph of the line that we find with the intercepts.