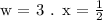Solution
Step 1:
Properties of Parallelograms Explained
1. Opposite sides are parallel. ...
2. Opposite sides are congruent. ...
3. Opposite angles are congruent. ...
4. Same-Side interior angles (consecutive angles) are supplementary. ...
5. Each diagonal of a parallelogram separates it into two congruent triangles. ...
6. The diagonals of a parallelogram bisect each other.
Step 2:
The diagonals of a parallelogram bisect each other.
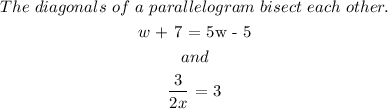
Step 3
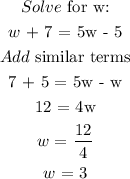
Step 4
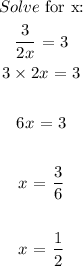
Final answer