Question

The half life of titanium - 44 , a radioactive isotope, is 63 years. If a substance starts out with 1000 kg of titanium- 44( round all the answers to the nearest hundredth of a kilogram or year) A) how much titanium- 44 will remain after 441 years ? B) how long will it be before there is only 1 kg of titanium- 44 ?

Answer 1

a)

Every 63 years, the amount of titanium halves.

441 years later means how many halving?

441/63 = 7 halving

We start off with 1000 and do 7 halving to get the amount of Titanium-44 after 441 years.

`\begin{gathered} 1000((1)/(2))^7 \\ =7.8125 \end{gathered}`

after 441 years, the amount of titanium remaining would be 7.8125 kg

b)

Let's find the point where the remaining titanium would be 1 kg.

That would be:

`1=1000((1)/(2))^t`

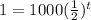
t is the time we are looking for. We can solve this using Ln(natural log):

`\begin{gathered} 1=1000((1)/(2))^t \\ 0.001=(1)/(2)^t \\ ln(0.001)=\ln ((1)/(2)^t) \\ \\ t=(\ln (0.001))/(\ln ((1)/(2))) \\ t=9.965 \end{gathered}`

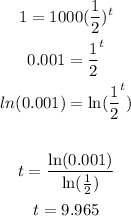
There is basically 9.965 halving. That would make the years approximately:

9.965 * 63 (half life) = 627.795 years (approx)