Question

How do i evaluate 8!4!/7!2!

Answer 1

Consider the following expression:

`(8!4!)/(7!2!)`

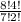
Remember that The factorial function is defined by the product:

`n!\text{ = }1\cdot2\cdot3\cdot\cdot\cdot\cdot\cdot\cdot(n-2)\cdot(n-1)\cdot n`

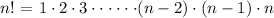
thus, according to this definition, the given expression can be expressed as:

`(8!4!)/(7!2!)\text{ = }\frac{(1\cdot2\cdot3\cdot4\cdot5\cdot6\cdot7\cdot8)\text{ (}1\cdot2\cdot3\cdot4\text{)}}{(1\cdot2\cdot3\cdot4\cdot5\cdot6\cdot7)(1\cdot2)}`

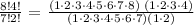
now, simplifying the previous expression we obtain:

`\text{= }(8)\text{ (}3\cdot4\text{) = }96`

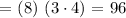
we can conclude that the correct answer is:

`\text{ }96`