Question

According to Wikipedia, as of November 1, 2021, 4,864 extrasolar planets have been identified. One of the closest multiple-planet solar systems to our own is around the star Gliese 876, about 15 light-years away, and it contains four planets. One takes 63.8 Earth days to revolve, at a distance of 3.07 x 107 kilometers from Gliese 876. Another planet takes 130 Earth days to revolve. How far is this second planet from Gliese 876?

Answer 1

Using kepler's law:

`(T1^2)/(r1^2)=(T2^2)/(r2^2)`

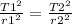
Where:

T1 = Planet's period of the first planet

T2 = Planet's period of the second planet

r1 = Average distance to Gliese of the first planet

r2 = Average distance to Gliese of the second planet

First, we need to do a conversion:

`\begin{gathered} T1=63.8days*(24h)/(1day)*(60min)/(1h)*(60s)/(1min)=5512320s \\ T2=130days(24h)/(1day)*(60m\imaginaryI n)/(1h)*(60s)/(1m\imaginaryI n)=11232000s \end{gathered}`

Now, solving for r2:

`\begin{gathered} r2=\sqrt{(r1^2\cdot T2^2)/(T1^2)} \\ r2=\sqrt{((3.07*10^7)^2(11232000)^2)/((5512320)^2)} \\ r2\approx62554858.93km \end{gathered}`

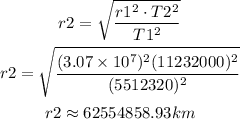
Answer:

62554858.93 km