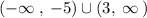Question

Solve the inequality |3x+3| + 3 > 15Write the answer in interval notation

Answer 1

Given the inequality:

`|3x+3|+3>15`

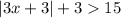
To solve the inequality,

step 1: Add -3 to both sides of the inequality.

Thus,

`\begin{gathered} |3x+3|+3-3>-3+15 \\ \Rightarrow|3x+3|>12 \end{gathered}`

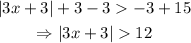
Step 2: Apply the absolute rule.

According to the absolute rule:

`\mathrm{If}\:|u|\:>\:a,\:a>0\:\mathrm{then}\:u\:<\:-a\:\quad \mathrm{or}\quad \:u\:>\:a`

Thus, from step 1, we have

`\begin{gathered} 3x+3<-12\text{ or 3x+3>12} \\ \end{gathered}`
`\begin{gathered} when \\ 3x+3<-12 \\ add\text{ -3 to both sides of the inequality} \\ 3x-3+3<-3-12 \\ \Rightarrow3x<-15 \\ divide\text{ both sides by the coefficient of x, which is 3} \\ (3x)/(3)<-(15)/(3) \\ \Rightarrow x<-5 \end{gathered}`
`\begin{gathered} when \\ 3x+3>12 \\ add\text{ -3 to both sides of the inequality} \\ 3x+3-3>12-3 \\ \Rightarrow3x>9 \\ divide\text{ both sides by the coefficient of x, which is 3} \\ (3x)/(3)>(9)/(3) \\ \Rightarrow x>3 \end{gathered}`

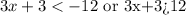
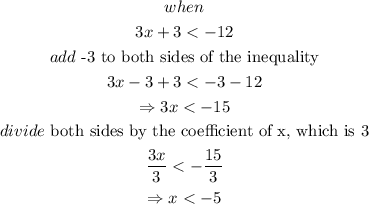
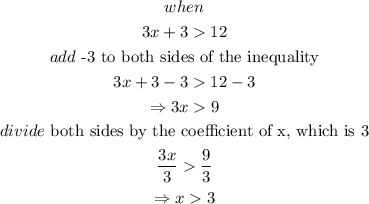
This implies that

`x<-5\quad \mathrm{or}\quad \:x>3`

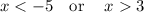
Hence, in interval notation, we have:

`\left(-\infty\:,\:-5\right)\cup\left(3,\:\infty\:\right)`