Given:
The output rate of Martinez family's sprinkler is 25L per hour and Green family's sprinkler is 35L per hour. The combined usage of sprinkler is 40 hours. The resulting water output is 1250L.
To find:
The number of hours each family used the sprinkler.
Solution:
Let Martinez family used sprinkler for x hours and Green family used sprinkler for y hours.
Since the combined usage of sprinklers is 40 hours. So,
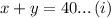
The output rate of Martinez family's sprinkler is 25L per hour and Green family's sprinkler is 35L per hour. The resulting water output is 1250L. So,
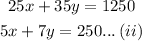
Multiply (i) by 7 and subtract from (ii), to get:

Now, we get x = 15, Put x = 15 in the equation (i):

Thus, x = 15, y = 25.