Question

The distance d (in inches) that a ladybug travels over time t(in seconds) is given by the function d (1) = t^3 - 2t + 2. Findthe average speed of the ladybug from t1 = 1 second tot2 = 3 seconds.inches/second

Answer 1

The Solution:

Given that the distance is defined by the function below:

`d(t)=t^3-2t+2`

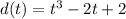
We are required to find the average speed of the ladybug from t=1 second to t=3 seconds in inches/second.

Step 1:

For t=1 second, the distance in inches is

`d(1)=1^3-2(1)+2=1-2+2=1\text{ inch}`

For t=3 seconds, the distance in inches is

`d(3)=3^3-2(3)+2=27-6+2=21+2=23\text{ inches}`

By formula,

`\text{ Average Speed=}\frac{\text{ distance covered}}{\text{ time taken}}`

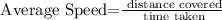
In this case,

Distance covered = change in distance, which is

`\text{ change in distance=d(3)-d(1)=23-1=22 inches}`

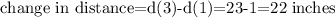
Time taken = change in time, which is:

`\text{ Change in time=t}_2-t_1=3-1=2\text{ seconds}`

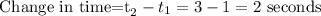
Substituting these values in the formula, we get

`\text{ Average Speed=}(22)/(2)=11\text{ inches/second}`

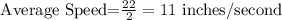
Therefore, the correct answer is 11 inches/second.