Let A be the event "person under 18" and B be the event "employed part-time". So, we need to find the following probability
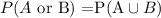
which is given by

Since the total number od people in the table is equal to n=4089, we have that

and
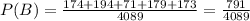
and
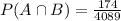
we have that
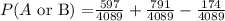
which gives
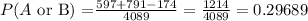
Therefore, the answer the searched probability is: 0.296