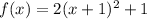To determine the vertex form of a parabola has equation:

where V(h,k) is the vertex of the parabola and 'a' is the leading coefficient.
From the question, we have that, the vertex is (-1, 1)
and the leading coefficient is a = 2
We substitute the vertex and the leading coefficient into the vertex form to
get:

The graph of this function is shown in the attachment.
Hence the equation of parabola is