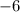Answer:
From the solution the largest possible integer value of x is;
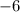
Step-by-step explanation:
Given the inequality;
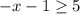
To solve, let's add 1 to both sides of the inequality;
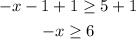
then let us divide both sides of the inequaty by -1.
Note: since we are dividing by a negative number the inequality sign will change.
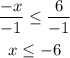
Therefore, From the solution the largest possible integer value of x is;