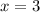1 Answer

Ask a Question

Steen · Answer 1 · 2023-02-11T17:10:57+0000

part 1 of the question is asking us to evaluate expressions to the power of 2. To do that let's remember the following:

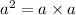
Ther is, when a number is elevated to second power it means we have to multiply that number by itself. For example:

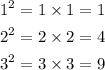
$\begin{gathered} 1^2=1*1=1 \\ 2^2=2*2=4 \\ 3^2=3*3=9 \end{gathered}$

In the second part of this question, we are asked to evaluate the square root of a number. Let's remember that the square root is the inverse operation of elevating a number to the second power. That is if we have:

$\sqrt[]{a}=b$

it means:

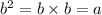
For example:

$\begin{gathered} \sqrt[]{1}=1 \\ \sqrt[]{4}=2 \\ \sqrt[]{9}=3 \end{gathered}$

The third part of the questions asks us to solve for "x" the following equation:

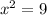
To do that we take square root on both sides:

$\sqrt[]{x^2}=\sqrt[]{9}$

Since the square root of a number to the second power is the same number and the square root of 9 is 3, we get:

0 Comments

Please log in or register to add a comment.

Please log in or register to answer this question.

1 Answer

0 Comments

Please log in or register to add a comment.

Other Questions