The slope of a line graph, we need to pick two point on the line.
It is better to pick points that meet the grids, so we can have its exactly coordinates.
Also, since we will need the y-intercept, one of the points can be this.
The y-intercept is the point where the line intercepts the y-axis, its x value is always 0, and we can see that it happens, in this case, at y = -8, so the point is (0, -8), and the y-intercept is -8.
Now, we can look at any other point. We can see that one point that meets the grids is the point at x = 3 and y = 1, so at point (3, 1).
The slope, m, can be, then, calculated as:
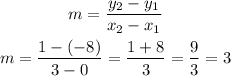
So, the slope is 3.
Answers:
Slope: 3
y-intercept: (0, -8)
The line in the slope-intercept form is:

where m is the slope and b is the y value of the y-intercept.
So, in this case, we have:
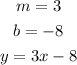
Which is exactly the same as the equation given, y = 3x - 8, so the equation given corresponds to the graph given.