Answer: the final volume of the balloon is 2.26 x 10^3 mL
Step-by-step explanation:
The question requires us to determine the new volume of a balloon, given the initial and final conditions.
The following information was provided by the question:
initial temperature = T1 = 24 °C = 297.15 K
initial volume = V1 = 443 mL
initial pressure = P1 = 745 mmHg = 0.980 atm
final temperature = T2 = -95 °C = 178.15 K
final pressure = P2 = 0.115 atm
To solve this problem, we'll need to apply the equation of ideal gases to calculate the number of moles of gas in the balloon, and then use this value and the final temperature and pressure provided to determine the final volume,
The equation of ideal gases can be written as:
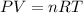
And we can rearrange it to calculate the number of moles:
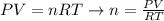
Applying the values provided by the question:
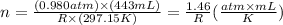
Now, we can rearrange the equation of ideal gases to calculate the volume:
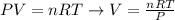
And, applying the values provided and the number of moles as calculated:

Therefore, the final volume of the balloon is 2.26 x 10^3 mL.