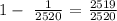Answer:
Step-by-step explanation:
Here, we want to get the probability that at least one man is assigned to the swing shift
From the question, 3 of the employees are assigned to the swing shift
Thus we have to calculate the probability of:
1 man , 2 men or 3 men
Mathematically, we have that as:
1 - p(all of the swing shift employees are women)
For the swing shift, for all them to be women, we will be selecting 3 out of 3 so the combination here is 3 C 3 which is 1
We now calculate the probability by dividing this value by the total number of possible ways
Mathematically, we have that as follows:
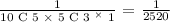
This is the probability of placing all of the women on the swing shift
So, the probability that at least 1 man is assigned will be: