Question

exercise after work, Albert (A) went running and Tanisha (T) walked for exercise. Their times and distances are showing in the graph below. How much as Albert running than Tanisha walking in miles per hour? Explain how you found your answer.

Answer 1

In the picture, there are two lines that graph distance versus time, so the slope of the line is teh rate or the speed of Albert or Tanisha.

We need to calculate the slope of each line. We can note that the two lines start in the origin point (0, 0), so:

`\begin{gathered} \text{For Albert, we can s}ee\text{ that the point (10, 1) is in the line, so:} \\ slopeofAlbert=m_A=(1)/(10)=0.1(miles)/(\min ute) \\ \text{For Tanisha, we can se}e\text{ thet the point (}20,\text{ 1) is in the line. so:} \\ slopeofTanisha=m_T=(1)/(20)=0.05(miles)/(\min ute) \end{gathered}`

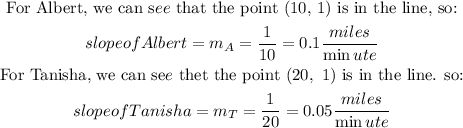
The different between the slopes (speed) is:

`\begin{gathered} m_A-m_T=0.1(miles)/(\min ute)-0.05(miles)/(\min ute)=0.05(miles)/(\min ute) \\ In\text{ miles/hours is:} \\ m_A-m_T=0.05(miles)/(\min ute)\cdot\frac{60\text{minutes}}{1\text{hour}}=3(miles)/(hour) \end{gathered}`

Albert goes 3 miles/hour faster than Tanisha