Let p be the number of pavilion seats and l be the number of lawn seats. Since there were sold 1400 tickets, we can write
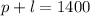
and since the total money was $32000, we can write
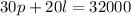
Then,we have the following system of equations
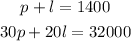
Solving by elimination method.
By multiplying the first equation by -30, we have an equivalent system of equation
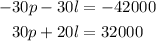
By adding these equations, we get
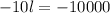
then, l is given by
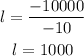
Now, we can substitute this result into the equation p+l=1400 and obtain
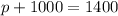
which gives
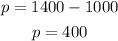
Then, How many tickets of each type were sold? 400 for pavilion seats and 1000 for lawn seats
How many pavilion seats were sold? 400 tickets