Question

A force of 30.ON is acted on a block of mass 15.0kg placed on a horizontal surface but the boxdoes not move. Calculate the magnitude of the frictional force between the surfaces when theapplied force is along the horizontal and when it makes angle 60° with the horizontal.

Answer 1

We will have the following:

1. In the first case, we will calculate the normal force:

Then, we will have that the fricion coefficient will be:

`\mu_f=(30.0N)/(147N)\Rightarrow\mu_f=(10)/(49)\Rightarrow\mu_f\approx0.20`

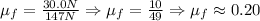
So the magnitude of the friccional force when applied horizontally will be of 30.0N.

2. And the magnitude when applied horizontal but the block is on an inclined surface of 60°, we will have that:

So, the friction force when the force is applied, is 15N.