Question

In the lab, Deandre has two solutions that contain alcohol and is mixing them with each other. Solution A is 10% alcohol and Solution B is 60% alcohol. He uses200 milliliters of Solution A. How many milliliters of Solution B does he use, if the resulting mixture is a 40% alcohol solution?

Answer 1

The percentage of alcohol of a solution i is given by the quotient:

`p_i=(v_i)/(V_i),_{}`

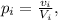
where v_i is the volume of alcohol in the solution i and V_i is the volume of the solution i.

From the statement of the problem we know that:

1) Solution A has 10% of alcohol, i.e.

`p_A=\frac{v_A_{}}{V_A}=0.1.\Rightarrow v_A=0.1\cdot V_A.`

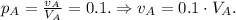
2) Solution B has 60% of alcohol, i.e.

`p_B=(v_B)/(V_B)=0.6\Rightarrow v_B=0.6\cdot V_B.`

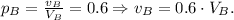
3) The volume of solution A is V_A = 200ml.

4) The resulting mixture must have a percentage of 40% of alcohol, so we have that:

`p_M=(v_M)/(V_M)=0.4.`

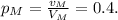
5) The volume of the mixture v_M is equal to the sum of the volumes of alcohol in each solution:

`v_M=v_A+v_{B\text{.}}_{}`

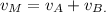
6) The volume of the mixtureVv_M is equal to the sum of the volumes of each solution:

`V_M=V_A+V_B\text{.}`

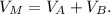
7) Replacing 5) and 6) in 4) we have:

`\frac{v_A+v_B}{V_A+V_B_{}}=0.4_{}\text{.}`

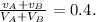
8) Replacing 1) and 2) in 7) we have:

`(0.1\cdot V_B+0.6\cdot V_B)/(V_A+V_B)=0.4_{}\text{.}`

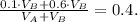
9) Replacing 3) in 8) we have:

`\frac{0.1\cdot200ml_{}+0.6\cdot V_B}{200ml_{}+V_B}=0.4_{}\text{.}`

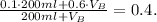
Now we solve the last equation for V_B:

`\begin{gathered} \frac{0.1\cdot200ml+0.6\cdot V_B}{200ml_{}+V_B}=0.4_{}, \\ \frac{20ml+0.6\cdot V_B}{200ml_{}+V_B}=0.4_{}, \\ 20ml+0.6\cdot V_B=0.4_{}\cdot(200ml+V_B), \\ 20ml+0.6\cdot V_B=80ml+0.4\cdot V_B, \\ 0.6\cdot V_B-0.4\cdot V_B=80ml-20ml, \\ 0.2\cdot V_B=60ml, \\ V_B=(60)/(0.2)\cdot ml=300ml. \end{gathered}`

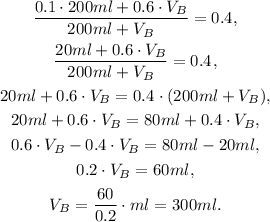
We must use 300ml of Solution B to have a 40% alcohol solution as the resulting mixture.

Answer: 300ml of Solution B.