We would begin by determining the slope of the line given;

To determine the slope, we would have to express the equation of the line in slope-intercept form as follows;

Therefore, we need to make y the subject of the equation as shown below;
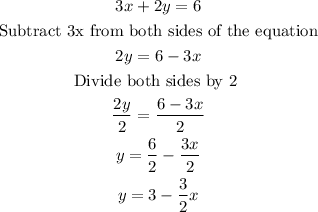
The equation in slope-intercept form appears as shown above. Note that the slope is given as the coefficient of x.
Note alo that the slope of a line perpendicular to this one would be a "negative inverse" of the one given.
If the slope of this line is
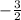
Then, the inverse would be

The negative of the inverse therefore is;
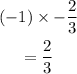
The answer therefore is option D