What is the equation for a line passing through (-2,5) perpendicular to y - 3x = 8

Question

asked Dec 17, 2023 177k views

1 Answer

Alen Giliana · Answer 1 · 2023-12-22T12:08:54+0000

Consider that the equation of a line with slope 'm' and y-intercept 'c' is given by,

Consider the given equation of line,

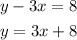
$\begin{gathered} y-3x=8 \\ y=3x+8 \end{gathered}$

Comparing the coefficient, it is found that the slope of the given line is 3,

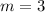
Let 's' be the slope of the line which is perpendicular to this line.

Consider that two lines will be perpendicular if their product of slopes is -1,

$\begin{gathered} m* s=-1 \\ 3* s=-1 \\ s=(-1)/(3) \end{gathered}$

So the slope of the perpendicular line is given by,

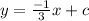
Now, it is given that this line passes through the point (-2,5), so it must satisfy the equation of the line,

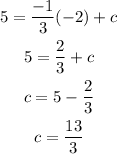
$\begin{gathered} 5=(-1)/(3)(-2)+c_{} \\ 5=(2)/(3)+c \\ c=5-(2)/(3) \\ c=(13)/(3) \end{gathered}$

Substitute the value of 'c' to get the final equation,

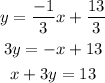
$\begin{gathered} y=(-1)/(3)x+(13)/(3) \\ 3y=-x+13 \\ x+3y=13 \end{gathered}$

Thus, the required equation of the perpendicular line is x + 3y = 13 .