Answer:
69 mph
Explanation:
The equation that represents this situation is:
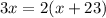
We are required to determine Brian's average speed on the return drive.
To do this, we first find the value of x.
![\begin{gathered} \text{Open the bracket} \\ 3x=2x+46 \\ \text{Subtract 2x from both sides} \\ 3x-2x=46 \\ x=46\; \text{mph} \end{gathered}]()
Using the value of x, we find the average speed on the return drive.
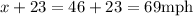
Therefore, the average speed on the return drive will be 69 mph.