Given the fucntion:
f(x) = tanx
Let's graph the function and input the correct values in the box.
• To find the y-intercept of the function, input 0 for x and solve:
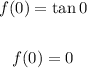
Therefore, the y-intercept is:
(0, 0)
• The period of the function:
The fundamental period of a tangent function is π.
Now, let's find points on the graph:
Therefore, the points are:
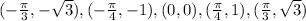
ANSWER:
The tangent function's period is π . The y-intercept of the function is (0, 0).
The points are:
![(-(\pi)/(3),-\sqrt[]{3}),(-(\pi)/(4),-1),(0,0),((\pi)/(4),1),((\pi)/(3),\sqrt[]{3})](https://img.qammunity.org/2023/formulas/mathematics/college/80r4ic60yoefkbrl2c7copwaaztmqgs2af.png)