Question

Segment AB and segment CD intersect at point E. Segment AC and segment DB are parallel.

Answer 1

To begin we shall sketch a diagram of the line segments as given in the question

As depicted in the diagram, line segment AC is parallel to line segment DB.

This means angle A and angle B are alternate angles. Hence, angle B equals 41 degrees. Similarly, angle C and angle D are alternate angles, which means angle C equals 56.

Therefore, in triangle EAC,

`\begin{gathered} \angle A+\angle C+\angle AEC=180\text{ (angles in a triangle sum up to 180)} \\ 41+56+\angle AEC=180 \\ \angle AEC=180-41-56 \\ \angle AEC=83 \end{gathered}`

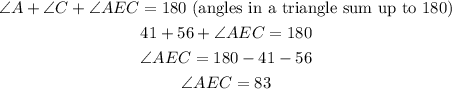
The measure of angle AEC is 83 degrees