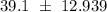Question

In a survey, 12 people were asked how much they spent on their child's last birthday gift. The results wereroughly bell-shaped with a mean of $39.1 and standard deviation of $17.4. Estimate how much a typical parentwould spend on their child's birthday gift (use a 99% confidence level). Give your answers to 3 decimal places.Express your answer in the format of ī + Error.$£ $

Answer 1

Given:

number of people (n) = 12

mean = 39.1

standard deviation = 17.4

99% confidence level

Using the confidence level formula, we can find the estimate of how much a typical parent would spend on their child's birthday:

`\begin{gathered} CI\text{ = x }\pm\text{ }\frac{z\varphi}{\sqrt[]{n}} \\ \text{where x is the mean} \\ z\text{ is the z-score at 99\% confidence interval} \\ \varphi\text{ is the standard deviation} \\ n\text{ is the number of people asked} \end{gathered}`

The z-score at 99% confidence level is 2.576

Substituting, we have:

`\begin{gathered} CI\text{ = 39.1 }\pm\text{ }\frac{2.576\text{ }*\text{ 17.4}}{\sqrt[]{12}} \\ =26.161\text{ and 52}.039 \end{gathered}`

Hence, a typical parent would spend between $26.161 and $52.039 or :

`39.1\text{ }\pm\text{ 12.939}`