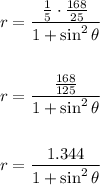Question

Construct a polar equation for the conic section with the focus at the origin and the following eccentricity and directrix.Conic Eccentricity Directrix1ellipsex= -75e =

Answer 1

In order to find the polar equation of the ellipse, first let's find the rectangular equation.

Since the directrix is a vertical line, the ellipse is horizontal, and the model equation is:

`((x-h)^2)/(a^2)+((y-k)^2)/(b^2)=1`

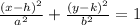
Where the center is located at (h, k), the directrix is x = -a/e and the eccentricity is e = c/a.

So, if the eccentricity is e = 1/5 and the directrix is x = -7, we have:

`\begin{gathered} (c)/(a)=(1)/(5)\rightarrow a=5c\\ \\ -(a)/(e)=-7\\ \\ (a)/((c)/(a))=7\\ \\ (a^2)/(c)=7\\ \\ (25c^2)/(c)=7\\ \\ 25c=7\\ \\ c=(7)/(25)\\ \\ a=5\cdot(7)/(25)=(7)/(5) \end{gathered}`

Now, let's calculate the value of b with the formula below:

`\begin{gathered} c^2=a^2-b^2\\ \\ (49)/(625)=(49)/(25)-b^2\\ \\ b^2=(25\cdot49)/(625)-(49)/(625)\\ \\ b^2=(24\cdot49)/(625)\\ \\ b^2=(1176)/(625) \end{gathered}`

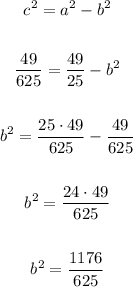
Assuming h = 0 and k = 0, the rectangular equation is:

`(x^2)/((49)/(25))+(y^2)/((1176)/(625))=1`

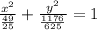
Now, to convert to polar form, we can do the following steps:

`\begin{gathered} (25x^2)/(49)+(625y^2)/(1176)=1\\ \\ 600x^2+625y^2=1176\\ \\ 600(r\cos\theta)^2+625(r\sin\theta)^2=1176\\ \\ 600r^2\cos^2\theta+625r^2\sin^2\theta=1176\\ \\ r^2(600\cos^2\theta+625\sin^2\theta)=1176\\ \\ r^2=(1176)/(600\cos^2\theta+625\sin^2\theta)\\ \\ r=\sqrt{(1176)/(600\cos^2\theta+625\sin^2\theta)}\\ \\ r=\sqrt{(1176)/(600+25\sin^2\theta)} \end{gathered}`

Another way of writing this equation in polar form is:

`r=(ep)/(1+\sin^2\theta)`

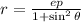
Where p is the distance between the focus and the directrix.

Since the foci are located at (±c, 0) = (±7/25, 0) and the directrix is x = -7, the distance is:

`p=7-(7)/(25)=(175)/(25)-(7)/(25)=(168)/(25)`

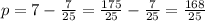
So the equation is:

`\begin{gathered} r=((1)/(5)\cdot(168)/(25))/(1+\sin^2\theta)\\ \\ r=((168)/(125))/(1+\sin^2\theta)\\ \\ r=(1.344)/(1+\sin^2\theta) \end{gathered}`