Given:
maximum = 2, minimum = -2
period = 2π/3
Find: the cosine function having those attributes stated above
Solution:
Cosine equations follow the pattern below:
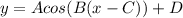
where A = amplitude, B = 2π/period, C = horizontal shift, and D = vertical shift.
Since the only given information is the period, let's calculate for the value of B.
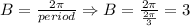
Out of the choices, only y = 2cos 3θ has the value of B which is 3. Hence, y = 2cos 3θ is the cosine function that has a maximum of 2, a minimum of –2, and a period of 2pi/3. (Option 3)