Question

For a science project, Sammy observed a chipmunk and a squirrel stashing acorns in holes. The chipmunk hid 3 acorns in each of the holes it dug. The squirrel hid 4 acorns in each of the holes it dug. They each hid the same number of acorns, although the squirrel needed 4 fewer holes. How many acorns did the chumpkin hide

Answer 1

Let x and y be the number of holes dug by the chipmunk and the squirrel, respectively.

Therefore, the number of hidden acorns by each animal is given by the equations below

`\begin{gathered} a_(chipmunk)=3x \\ a_(squirrel)=4y \end{gathered}`

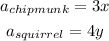
On the other hand, since the squirrel needed 4 fewer holes, and the number of hidden acorns is the same

`\begin{gathered} y=x-4 \\ and \\ a_(chipmunk)=a_(squirrel) \end{gathered}`

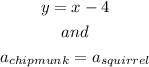
Thus,

`\begin{gathered} \Rightarrow3x=4y \\ \Rightarrow3x=4(x-4) \\ \Rightarrow3x=4x-16 \\ \Rightarrow x=16 \end{gathered}`

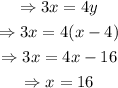
Hence,

`\Rightarrow16*3=48`

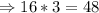
The chipmunk hid 48 acorns.