w=2
All real numbers are solutions
1) In this question, let's solve each equation, and then we can check whether there are solutions, which one would be.
2) Let's begin with the first one, top to bottom
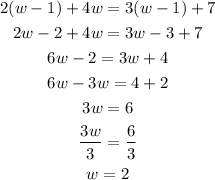
Note that we distributed the factors outside the parenthesis over the terms inside.
So for the first one, we can check w=2
3) Moving on to the 2nd equation, we can state:
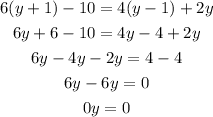
So, there are infinite solutions for this equation, or All real numbers are solutions