Question

Basically, the question is asking to solve a problem about rectangular prisms. It says the the shape of one box, with (h) height in feet, has a volume defined by the function:V(h) = h (h-5)(h-6)It says to graph the function. What is the maximum volume for the domain 0

Answer 1

The function representing the volume of the rectangular prism is given to be:

`V(h)=h(h-5)(h-6)`

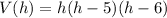
Since we are expected to find the volume using the graph, we can prepare a table of values for the function using values of h as integers from 1 - 5, such that:

`\begin{gathered} At\text{ }h=1 \\ V(1)=1(1-5)(1-6)=-4*-5=20 \end{gathered}`

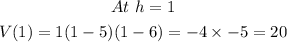
The completed table is shown below:

Hence, we can plot these points on a graph using a graphing calculator for ease of work. This is shown below:

The maximum volume of the prism is represented by the highest point on the graph. The graph's highest point is at:

`h=1.811`

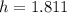
The corresponding value for the volume as can be seen on the graph is:

`V=24.193`

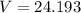
This is the maximum volume of the prism.

To the nearest cubic foot, the maximum volume of the rectangular prism is 24 cubic feet.