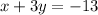Question

3. Find the equation of a line passing through (5,-6) parallel to : x + 3y = 8

Answer 1

The general slope intercept form is : y = m * x + b

Where m is the slope and b is y - intercept

Given the equation of the line : x + 3y = 8

Write the equation in slope intercept form to find the slope of the line

so, solve for y :

`\begin{gathered} x+3y=8 \\ 3y=-x+8 \\ \\ y=-(1)/(3)x+(8)/(3) \end{gathered}`

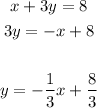
So, the slope of the given line = -1/3

The parallel lines have the same slope

so, the slope of the required line = -1/3

And the equation will be :

`y=-(1)/(3)x+b`

Find the value of b using the given point ( 5 , -6 )

When x = 5 , y = -6

`\begin{gathered} -6=-(1)/(3)\cdot5+b \\ -6=-(5)/(3)+b \\ -6+(5)/(3)=b \\ \\ b=-(13)/(3) \end{gathered}`

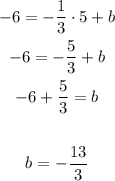
So, the equation of the line will be :

`y=-(1)/(3)x-(13)/(3)`

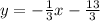
it can be written as : 3y = -x - 13

`x+3y=-13`