Solution:
Given:
The table of values is given:
From the table,
We see the data is a linear function. This is because a linear function has domain values at regular intervals.
Also, the linear equation can be formed as shown below, indicating it is a linear function.
Considering two points, (3,1) and (1,2)
where,
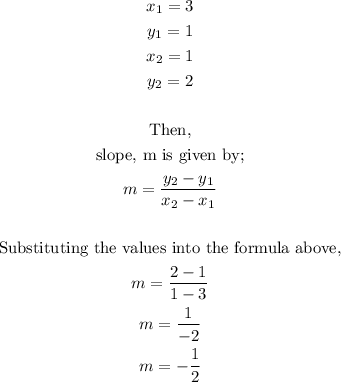
A linear equation is of the form;
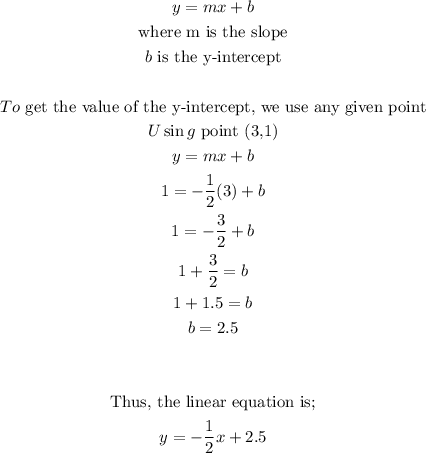
From the above, has confirmed it is a linear function and not an exponential function, we can deduce that;
a) The function is not an exponential function.
b) The domain values (x-values) are at regular intervals
c) The range values (y-values) have a common difference of 1
Therefore, the correct answer is OPTION A