Question

What is the equation of the line that passes through the origin and is perpendicular to the line 4x+3y=6

Answer 1

The Equation of a Line

The slope-intercept form of a line can be written as:

y = mx + b

Where m is the slope of the graph of the line and b is the y-intercept.

In the specific case where the line passes through the origin (0,0), we can find the value of b by substituting x=0 and y=0:

0 = m(0) + b

Solving for b:

b = 0.

Thus, the equation of the line reduces to:

y = mx

We only need to find the value of the slope.

That is where we need the second data. Our line is perpendicular to the line of equation 4x + 3y = 6.

Solving for y:

`y=-(4)/(3)x+2`

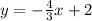
The slope of the second line is -4/3.

We must recall that if two lines of slopes m1 and m2 are perpendicular, then:

`m_1\cdot m_2=-1`

Substituting the value of m1 and solving for m2:

`\begin{gathered} -(4)/(3)\cdot m_2=-1 \\ m_2=(3)/(4) \end{gathered}`

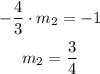
The slope of our line is 3/4 and the required equation is:

`y=(3)/(4)x`

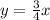
From this last equation, we need to find the general form of the line.

Multiply both sides of the equation by 4:

4y = 3x

Subtract 3x on both sides:

4y - 3x = 0

Reorder:

-3x + 4y = 0