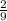Answer:
The fraction form of the repeating decimal is;
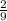
Step-by-step explanation:
We want to express the repeating decimal 0.2 (2 repeating) as a fraction.
let x represent the fraction;
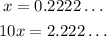
Then subtract x from 10x;
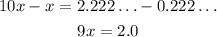
Then we can divide both sides by the coefficient of x;
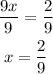
Therefore, the fraction form of the repeating decimal is;