Question

4 ft 12 ft The pitch of the roof is

Answer 1

As shown : in the figure

The pitch of the roof is the angle between the roof and the horizontal line

As shown we have a right angle triangle

The opposite side to the angle = 4 ft

And the adjacent side to the angle = 12 ft

According to the given sides, we will calculate the angle using tan function

So, let the angle = x

So,

`\begin{gathered} \tan x=(opposite)/(adjacent) \\ \\ \tan x=(4)/(12)=(1)/(3) \\ \\ x=\tan ^(-1)(1)/(3)\approx18.435^o \end{gathered}`

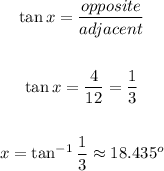
So, the pitch angle of the roof = 18.435

instead of writing the angle , just we will write the slope = rise/run

So, the pitch of the roof = 1/3