The table given showed the discrete probability distribution for random variables 3 to 6 and their corresponding probability except for the probability of 4
It should be noted that for a probability distribution, the cummulative probabibility (that is the sum of all the probability) must be equal to one.
This means that
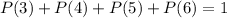
From the given table, it can be seen that
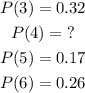
Then, p(4) is calculated below
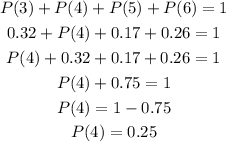
Hence, P(4) is 0.25