Let's use the variable x to represent the length of the paper and y to represent the width of the paper.
If there are margins of 1 inch along the sides and 3 inches along the top and bottom, and the area with printer matter is 27 in², we can write the equation:
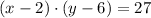
Solving for x, we have:
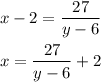
Then, the equation that we want to minimize is the area equation, so:
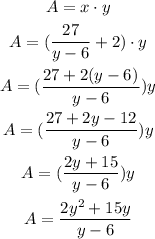
The critical points of this function are:
y = 0 (one of the roots)
y = -15/2 (one of the roots)
y = 6 (function is not defined)