Question

Suppose that the number of bacteria in a certain population increases according to a continuous exponential growth model. The sample of 2100 bacteria selected from this population reach the size of 2249 bacteria in two and a half hours. Find the hourly growth rate parameter.This is a continuous exponential growth model.Write your answer as a percentage. Do not round any intermediate computations, and round your percentage to the nearest hundredth.

Answer 1

In this problem, we have a continuous exponential growth model

so

the equation is of the form

`y=a(e)^(kt)`

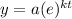
where

a is the initial value ------> a=2,100

y is the number of bacteria

x ----> number of hours

so

`y=2,100(e)^(kt)`

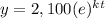
For x=2.5 hours, y=2,249 bacteria

substitute

`2,249=2,100(e)^((2.5k))`

solve for k

apply ln both sides

`\ln ((2,249)/(2,100))=2.5k\cdot\ln (e)`

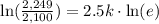
k=0.0274

convert to percentage

k=2.74%