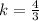Question

Hey everybody! Can somebody help me solve this problem? I don't need a big explanation just the answer and a brief explanation on how you get it! Look at photo for problem.

Answer 1

Given the ordered pairs:

(-12, -16), (-3, -4), (0, 0), (9, 12)

Let's say that the first coordinate corresponds to x, and the second one corresponds to y. Then, the constant of variation k relates x and y as:

`y=k\cdot x`

Using the ordered pairs:

`\begin{gathered} -16=-12k\Rightarrow k=(4)/(3) \\ -4=-3k\Rightarrow k=(4)/(3) \\ 0=0\cdot k\text{ (this means that it is correct)} \\ 12=9k\Rightarrow k=(4)/(3) \end{gathered}`

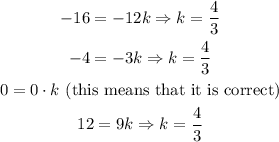
We conclude that the constant of variation is:

`k=(4)/(3)`