Question

Solve p(x+q)^4=r for x

Answer 1

Given the following equation:

`p\mleft(x+q\mright)^4=r`

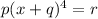
You can solve for the variable "x" as following:

1. You need to apply the Division property of equality by dividing both sides of the equation by "p":

`\begin{gathered} (p\mleft(x+q\mright)^4)/(p)=(r)/(p) \\ \\ \mleft(x+q\mright)^4=(r)/(p) \end{gathered}`

2. Remember that:

`\sqrt[n]{a^n}=a`

Then:

`\begin{gathered} \sqrt[4]{(x+q)^4}=\sqrt[4]{(r)/(p)} \\ \\ x+q=\sqrt[4]{(r)/(p)} \end{gathered}`

3. Now you have to apply the Subtraction property of equality by subtracting "q" from both sides of the equation:

`\begin{gathered} x+q-(q)=\sqrt[4]{(r)/(p)}-(q) \\ \\ x=\sqrt[4]{(r)/(p)}-q \end{gathered}`

The answer is:

`x=\sqrt[4]{(r)/(p)}-q`